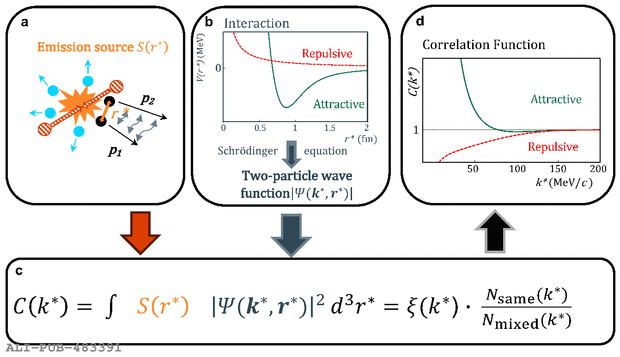
Schematic representation of the correlation method. a, A collision of two protons generates a particle source $S(\rs)$ from which a hadron--hadron pair with momenta $\bm{p}\bf{_1}$ and $\bm{p}\bf{_2}$ emerges at a relative distance $r^*$ and can undergo final-state interaction before being detected Consequently, the relative momentum \ks is either reduced or increased via an attractive or a repulsive interaction, respectively b, Example of attractive (green) and repulsive (dotted red) interaction potentials, V(\rs), between two hadrons, as a function of their relative distance Given a certain potential, a non-relativistic Schr\"odinger equation is used to obtain the corresponding two-particle wave function, $\psi(\bm{k}^*,\bm{r}^*)$ c, The equation of the calculated (second term) and measured (third term) correlation function C(\ks), where $N_{\mathrm{same}}(\ks)$ and $N_{\mathrm{mixed}}(\ks)$ represent the \ks distributions of hadron--hadron pairs produced in the same and in different collisions, respectively, and $\xi(\ks)$ denotes the corrections for experimental effects d, Sketch of the resulting shape of C(\ks) The value of the correlation function is proportional to the interaction strength It is above unity for an attractive (green) potential, and between zero and unity for a repulsive (dotted red) potential